Comparing linear and exponential growth
Many things grow, but not all things grow in the same way. There are two basic ways for something to grow: linearly, and exponentially. When something grows linearly it grows repeatedly by the same amount; when something grows exponentially it grows repeatedly by the same proportion.
Imagine that you have a bank savings account that pays no interest, and at the end of each year you add $10 to the account. If you start with $100 in the account, after one year the account will hold $110; after two years it will hold $120 dollars; after three years it will hold $130, and so on. This is linear growth; it grows repeatedly by the same amount, $10 in this example.
Now consider that you have a bank savings account that pays 10% interest per year: at the end of each year 10% of the amount in the account at that time is added to the account by the bank. If you start with $100 in it, after a year the bank will add the interest and the account will have $110; after two years it will have $121; after three years it will have $133.10, and so on. This is exponential growth; it grows repeatedly by the same proportion, 110% in this example.
Let's see the progress of the outcomes in a table:
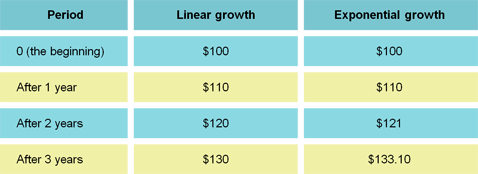
After three years exponential growth has clearly given a bigger outcome than linear growth, but not by much: $3.10. Over a longer period of growth the difference becomes disproportionately much greater; the following table gives values out to twenty-one years.
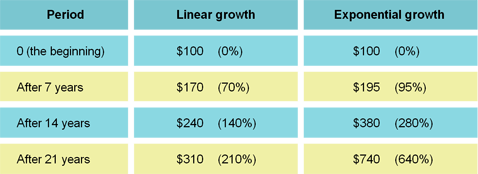
After twenty-one years, the linearly growing $100 has grown by slightly more than three times, while the exponentially growing $100 has grown by more than seven times.
 choose the future!
choose the future!
Write a comment