Many things grow, but not all things grow in the same way. There are two basic ways for something to grow: linearly, and exponentially. When something grows linearly it grows repeatedly by the same amount; when something grows exponentially it grows repeatedly by the same proportion.

If something grows constantly, it will eventually double its original size. The period of time it takes to double is the doubling period; the doubling period is a useful concept when examining exponential growth.

To examine some properties of exponential growth and periodic doubling, consider a standard chess board, with eight squares on each side for a total of sixty-four squares. We place a single grain of wheat on the first square, two grains of wheat on the second square, four grains of wheat on the third square, eight grains of wheat on the fourth square; and so on, placing twice as many grains of wheat on each square as we did on the previous square.

Because the number of grains grows by the same proportion (it doubles, 200%), repeatedly (every square) the growth of the number of grains is exponential. Our doubling period is one square of the chess board; when we have finished placing the wheat we will have performed sixty-three doublings of the single grain of wheat on the first square.

So, how much wheat have we got? This is where we find out how surprising and counter-intuitive exponential growth is. You probably have a mental picture of a few sparse wheat grains at one end of the chess board building to a thick layer at the other end of the board. In fact, the number of grains 'on' the chess board is 4,611,686,018,427,387,903, if none get spilled.

This is obviously a huge number, so big as to make no real meaningful mental impression. A more evocative value is that it is approximately four-hundred times the world's 1990 harvest of wheat, and is probably more wheat than has ever been grown and harvested by humanity.

This is the first important point about exponential growth:

Exponential growth produces huge numbers unexpectedly quickly.

Let's go back to the beginning of the process of placing the wheat grains on the chess board; here is a table of the grains on the first few squares, and the progressive totals for the whole chess board.

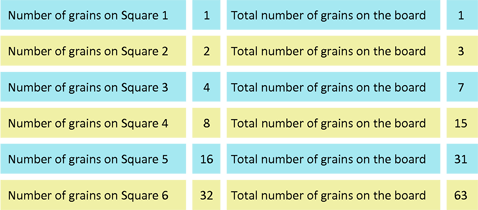
Look at these numbers and you will see that when a new square gets completed, the total of all the grain on the board is one grain less than twice the number on that square. For example, when square six has been filled it has 32 grains on it; twice 32 is 64, less one grain gives 63, which is the total of all the grain on the board at that point. It's safe to assume that this pattern will hold for the whole chess board.

After just the first few squares, that one grain less in the total becomes insignificant. So you can say that every time you complete a square, that square has half of all of the grain that is currently on the chess board; that is, that square has as much on it as all of the previous squares added together. For example, when square 6 has been completed it has 32 wheat grains on it; squares 1 to 5 have 31 wheat grains (1 + 2 + 4 + 8 + 16) between them, so square 6 has very nearly half of all the grain on the board at that point.

This happens no matter which square of the chess board we look at. If we were to extend the chess board to more that 64 squares, it would happen no matter how big we made it: the last square completed would always be equal to all of the previous squares added together. This is true for the doubling period of anything that is growing exponentially.

This is the second important point about exponential growth:

The growth that happens in any doubling period is equal to all of the growth that has ever happened before.

Now let's go to the end of the process of placing the wheat grains on the chess board and look at what happens in the last few squares, when the chess board is nearly completed.

Our second important point about exponential growth tells us that the last completed square, square 64, has half of all of the grain on it. Remembering that each square has half as much grain on it as the following square has, we know that square 63 has a quarter of all of the grains on it, square 62 has one-eighth of all of the wheat grains on it, and so on.

Here is a table of the proportions of the grain on the last five squares.

The last five squares, 60 to 64, will have 96.9% of all of the grain on the chess board.

When we have completed 59 of the 64 squares on the chess board we have completed most of the squares on the board (92%), but we have only placed 3.1% of the grain on the board; the other 96.9% will go on the last five squares.

Notice that the last five doublings don’t depend on how many doublings have gone before; no matter how many doublings have happened as something grows exponentially, the last five doublings will always represent 96.9% of the total.

This is the third important point about exponential growth:

Most of the growth happens very fast, at the very end of the process.

Here are our three important points about exponential growth:

• Exponential growth produces huge numbers unexpectedly quickly.

• The growth that happens in a doubling period is equal to all the growth that has ever happened before it.

• Most of the growth happens very fast, at the very end of the process.

This page is linked from:

economic growth  

exponential growth

resource recycling and economic growth

real world exponential growth

blog post: intergenerational revenge

Agree? Disagree? Make a comment!  (Comments are moderated)